NOTES:
Inverse Function: a function that reverses another function. If the function f applied to an input x gives a result of y, then applying the inverse function g to y gives the result x, and vice versa.
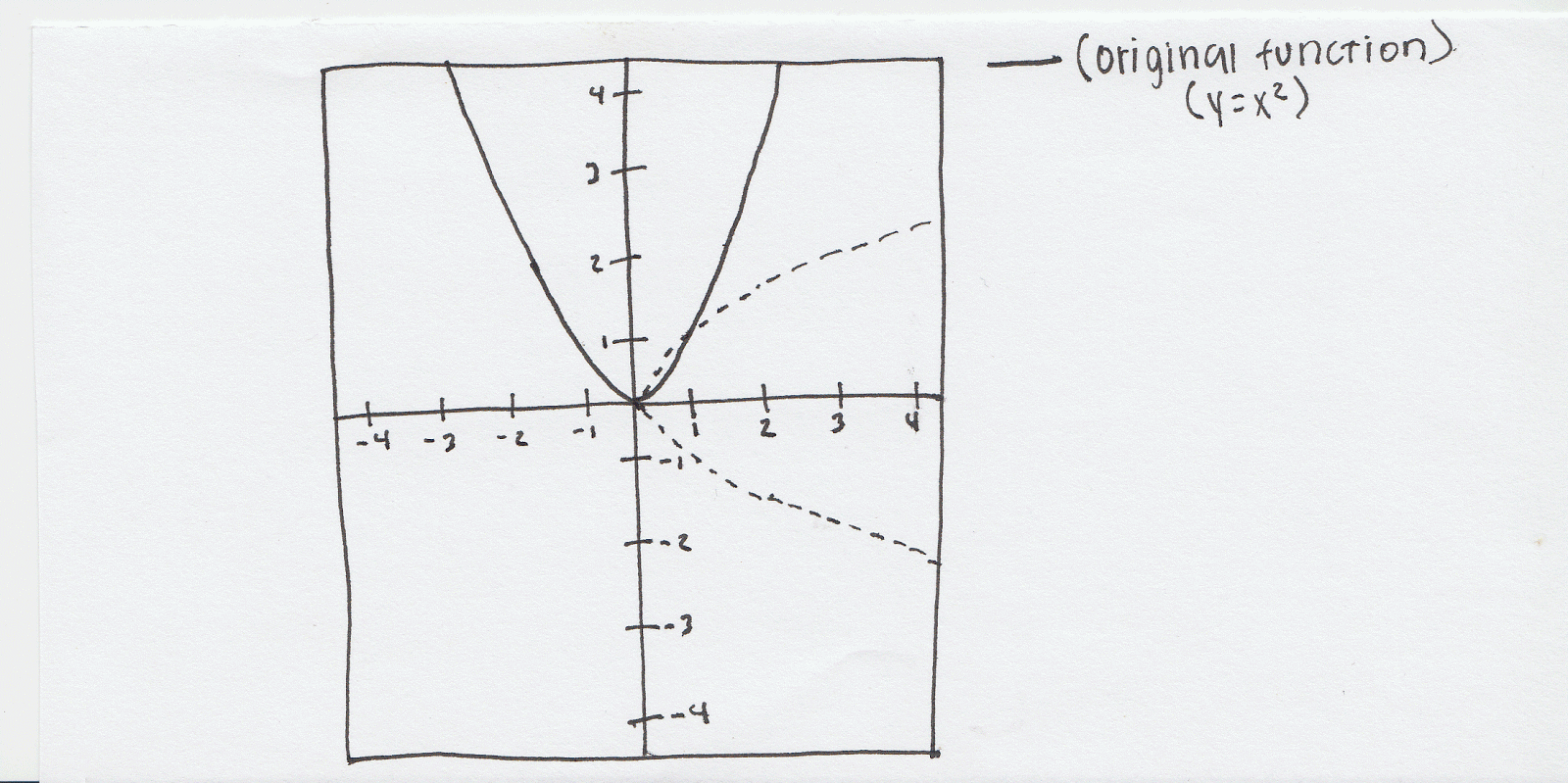
Problem 1: Determine graphically if a function has an inverse which is also a function.
If a function has in inverse function, the reflection of that original function in the identity line, y=x, will also be a function. It will pass the vertical line test for functions.
Problem 2:
This is just a graphic representation of an inverse function.
PROBLEM 1
PROBLEM 2
REFLECTION
These can be confusing sometimes, mostly problems like number 2. To graph all you do is switch x and y. I think it is easy to do the vertical line test.
ADDITIONAL RESOURCES
http://www.purplemath.com/modules/invrsfcn.htm